Particle behavior at the nuclear and subatomic levels arranges quantum mechanics as a part of physical science. It challenges our traditional comprehension of physical science. It presents a reality where particles can exist in various states simultaneously, where the demonstration of perception impacts the condition of a molecule, and where trapped particles momentarily influence each other, paying little heed to remove them. This blog will dig into the essentials of quantum mechanics, investigating its key standards, verifiable turn of events, exploratory proof, and suggestions for different areas of science and innovation.
1. Verifiable Improvement of Quantum Mechanics
The excursion of quantum mechanics started in the mid-twentieth century when traditional physical science couldn’t make sense of specific peculiarities. Key achievements in its improvement include:
1.1. Blackbody Radiation
The old-style hypothesis of blackbody radiation, which portrays an ideal blackbody’s discharge of electromagnetic radiation, couldn’t explain the noticed range. Max Planck settled this in 1900 by recommending that energy is quantized, meaning it must be discharged or consumed in discrete sums called quanta.
1.2. The Photoelectric Impact
In 1905, Albert Einstein made sense of the photoelectric impact, where electrons are produced from a material when light sparkles. He recommended that light comprises particles called photons, each conveying a quantum of energy. This gave additional proof that energy is quantized.
1.3. The Bohr Model of the Molecule
In 1913, Niels Bohr presented a model of the molecule with quantized energy levels. He recommended that electrons circle the core in unambiguous, stable circles and can bounce between these circles by retaining or discharging photons. This model made sense of the phantom lines of hydrogen.
1.4. Wave-Molecule Duality
In 1924, Louis de Broglie recommended that particles, similar to electrons, show both wave-like and molecule-like properties. This wave-molecule duality was affirmed by the twofold cut explore, which showed that electrons make an obstruction design when going through two cuts, a trait of waves.
1.5. The Schrödinger Condition
Erwin Schrödinger, in 1926, fostered a wave condition that portrays how the quantum condition of an actual framework changes over the long haul. The Schrödinger condition is a focal condition in quantum mechanics. It structures the reason for understanding how particles behave at the quantum level.
2. Key Standards of Quantum Mechanics
Quantum mechanics is based on a few central rules that separate it from old-style material science:
2.1. Superposition
Superposition is the rule that a quantum framework can exist in numerous states simultaneously. For instance, a molecule can be in both 0 and 1 simultaneously. When an estimation is made, the framework falls to one of the potential states.
2.2. Trap
Trap is a peculiarity in which the quantum conditions of at least two particles become connected to such an extent that the condition of one molecule quickly influences the condition of the other, no matter what the distance between them. This non-neighborhood connection has been tentatively affirmed and difficult old-style ideas of region and causality.
2.3. Wave-Molecule Duality
Wave-molecule duality is the idea that particles, such as electrons and photons, show both wave-like and molecule-like properties. This duality is shown by peculiarities like diffraction and obstruction, which are normal for waves, and the photoelectric impact, which is normal for particles.
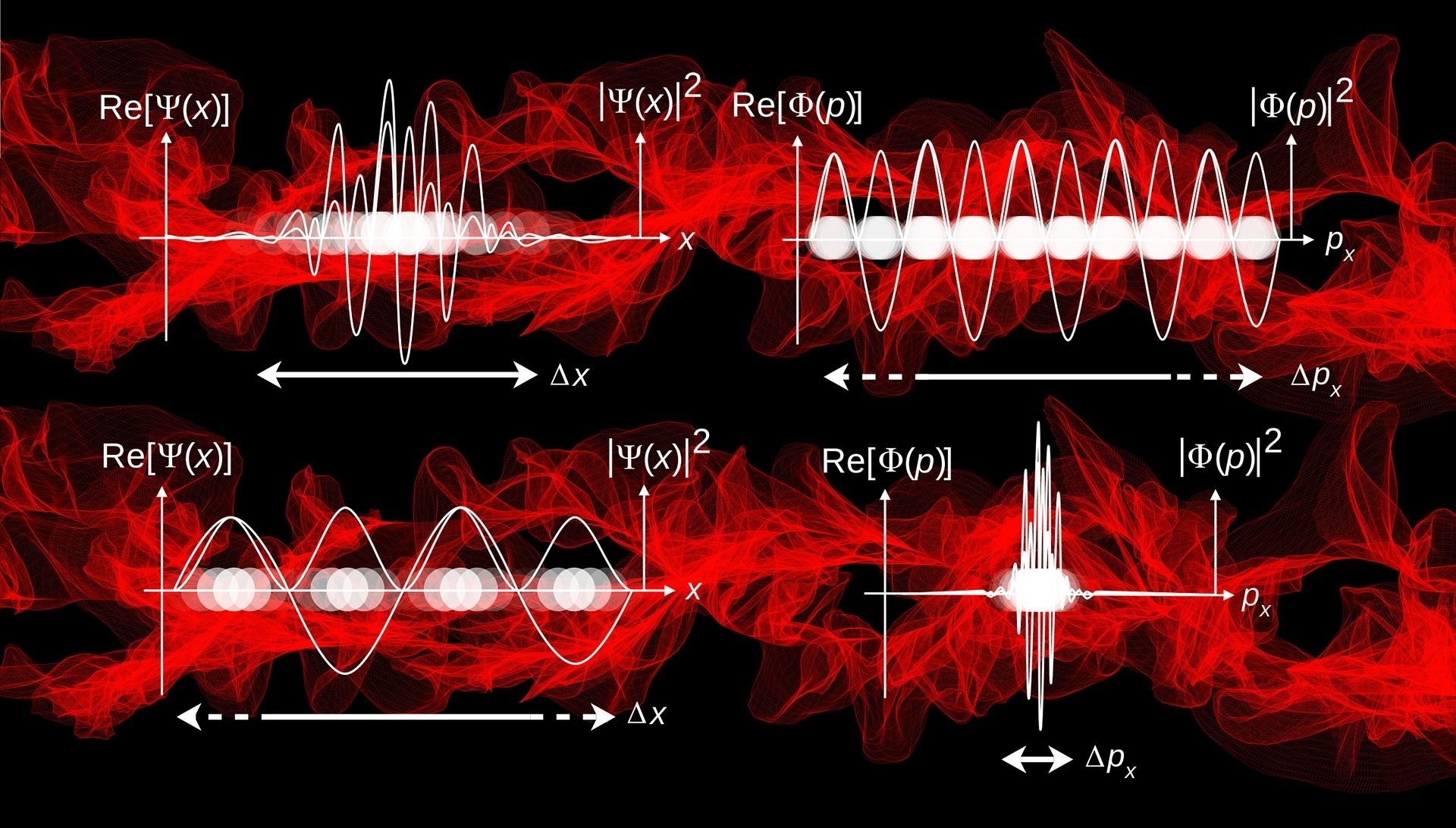
2.4. Heisenberg’s Vulnerability Guideline
Heisenberg’s vulnerability guideline states that it is difficult to gauge a molecule’s specific position and force with outright accuracy. The more precisely one property is estimated, the less precisely the other can be known. This guideline features the innate limits of estimation at the quantum level.
2.5. Quantum Burrowing
Quantum burrowing is a peculiarity in which particles can overcome energy hindrances that would be unfavorable in traditional material science. This happens because particles are wave-like, permitting them to exist in districts of rooms that are traditionally prohibited.
3. Numerical System of Quantum Mechanics
Quantum mechanics is figured out utilizing a numerical system that incorporates wave capabilities, administrators, and the Schrödinger condition:
3.1. Wave Capabilities
The wave capability is a numerical portrayal of the quantum condition of a framework. It contains all the data about the framework and is commonly addressed by the Greek letter ψ (psi). The square of the wave capability’s extent gives the likelihood thickness of tracking down a molecule in a specific state.
3.2. Administrators
Administrators are numerical elements that follow up on wave capabilities to extract actual data about a framework. Normal administrators incorporate the position administrator, force administrator, and Hamiltonian administrator, which relate to the framework’s absolute energy.
3.3. The Schrödinger Condition
The Schrödinger condition is a halfway differential condition that portrays how the wave capability of a quantum framework develops over the long run. It comes in two structures: the time-subordinate Schrödinger condition, which applies to frameworks whose quantum state changes over the long run, and the time-free Schrödinger condition, which applies to frameworks in a fixed state.
4. Trial Proof for Quantum Mechanics
Quantum mechanics is upheld by various tests that show its standards and forecasts:
4.1. The Twofold Cut Analysis
The twofold cut try, first performed by Thomas Youthful with light and later with electrons, demonstrates how particles can behave in a wave-like way. When particles go through two cuts, they make an obstruction design on a finder screen, demonstrating wave-like impedance.
4.2. Chime’s Hypothesis and Ensnarement Tests
Chime’s hypothesis, proposed by physicist John Ringer, provides a method for testing quantum mechanics’ expectations against old-style speculations of nearby authenticity. Tests testing Chime’s imbalances have affirmed quantum mechanics’ expectations, exhibiting the truth of the trap.
4.3. The Harsh Gerlach Trial
The Harsh Gerlach try exhibited the quantization of rakish force by passing light emission iotas through a non-uniform attractive field. The iotas were diverted into discrete spots on an identifier screen, proving the presence of quantized turn states.
4.4. The Casimir Impact
The Casimir impact is an actual power emerging from the quantum vacuum variances between two firmly dispersed metal plates. It gives exploratory proof of the truth of vacuum changes anticipated by the quantum field hypothesis.
5. Ramifications of Quantum Mechanics
Quantum mechanics has significant ramifications for different areas of science and innovation:
5.1. Quantum Figuring
Quantum figuring uses the standards of superposition and ensnarement to perform calculations that are infeasible for old-style PCs. Quantum calculations, like Shor’s and Grover’s, offer dramatic speedups for issues in cryptography, search, and enhancement.
5.2. Quantum Cryptography
Quantum cryptography utilizes the standards of quantum mechanics to make secure correspondence channels. Quantum critical circulation (QKD) conventions, like BB84, permit two gatherings to impart a mystery key to security ensured by the laws of physical science.
5.3. Quantum Instant transportation
Quantum instant transportation is a cycle by which the quantum condition of a molecule is sent starting with one area and then onto the next, utilizing entrapment and old-style correspondence. This cycle has been tentatively shown and has likely applications in quantum correspondence and calculation.
5.4. Quantum Sensors
Quantum sensors exploit quantum rationality and ensnarement to accomplish high-accuracy estimations. Applications incorporate nuclear timekeepers, magnetometers, and gravitational wave indicators, which have improved awareness compared with old-style sensors.
6. Difficulties and Open Inquiries in Quantum Mechanics
Notwithstanding its triumphs, quantum mechanics faces a few difficulties and open inquiries:
6.1. The Estimation Issue
The estimation issue concerns how and why the wave capability implodes during an estimation. Different translations of quantum mechanics, like the Copenhagen understanding, many-universes translation, and goal breakdown speculations, offer various answers for this issue.
6.2. Quantum Gravity
Quantum mechanics and general relativity are the two mainstays of present-day physical science. Yet, they are fundamentally contrary. Fostering a hypothesis of quantum gravity that brings together these two systems is the most significant open inquiry in material science.
6.3. Decoherence
Decoherence is the cycle by which a quantum framework loses its soundness and acts more traditionally because of cooperation with its current circumstances. Understanding and relieving decoherence is significant for the advancement of pragmatic quantum innovations.
Conclusion
Quantum mechanics has essentially changed how we interpret the actual world, uncovering a domain where particles can exist in numerous states all the while, where the demonstration of perception influences the framework, and where trapped particles stay associated across huge distances. Its standards and expectations have been affirmed by various investigations, and it has significant ramifications for different areas of science and innovation. As we investigate and foster quantum mechanics, we hope to reveal significantly more profound bits of knowledge about the idea of the natural world and open new advances that will change our reality.
Source: Internet & AI